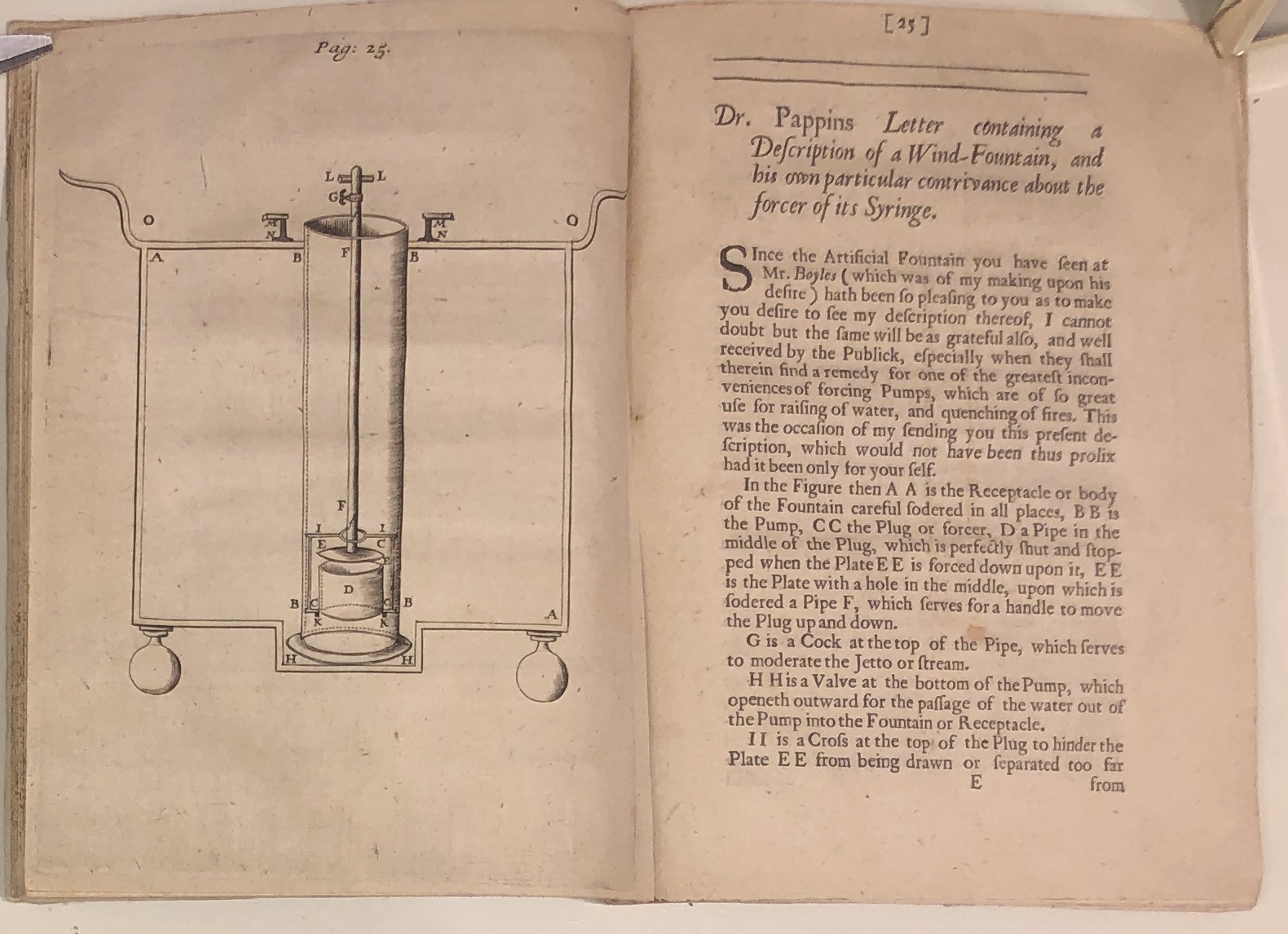
Description

HOOKE, Robert. Lectures De Potentia Restitutiva, or of Spring. Explaining the Power of Springing Bodies. To which are added some Collections, viz. A description of Dr. Pappin’s wind-fountain and force-pump. Mr. Young’s observations concerning natural fountains. Some other considerations concerning that subject. Captain Sturmy’s remarks of a subterraneous cave and cistern. Mr. G.T. observations made on the Pike of Teneriff, 1674. Some reflections and conjectures occasioned thereupon. A relation of the late eruption in the Isle of Palma. London: Printed for John Martyn, Printer to the Royal Society, at the Bell in St. Pauls Church-Yard, 1678.

First edition, very rare, containing ‘Hooke’s law’, that stress is proportional to strain, and the first kinetic theory of gases. “This tract is of fundamental importance” (Keynes, Bibliography, p. 40). Hooke’s attempts to develop a spring-regulated watch had led him in the 1660s to the secret of springs, which he had revealed in the form of an anagram ‘ceiiinosssttuu’ in ‘Helioscopes’ (1676), but it was only in the present work that he revealed the solution to his anagram, ‘Ut tensio sic vis’, that is, ‘The power of any spring is in the same proportion with the tension thereof’.

“Hooke’s discourse on springs, which was published as ‘Lectures De Potentia Restitutiva’, was much more than a description of the way that springs and other elastic bodies behave under tension. What he was trying to do in his lectures was to explore the fundamental causes of elasticity, and in doing so he put forward a theory of matter that was different to anything that had been proposed before, and similar in many respects to the kinetic theory of gases that was not generally accepted until the 1860s. Hooke began with a brief history of his theory, claiming to have discovered it eighteen years earlier, when he first proposed his spring-regulated watch, ‘but designing to apply it to some particular use, I omitted the publishing thereof’. Then he taught his readers how to make a simple spring balance by coiling a length of steel, brass or iron wire into a helix around a cylinder, suspending the resulting coil on a nail, hanging weights of different sizes from its lower end and measuring the resulting extension of the spring. They would find that if a one-pound weight stretched the spring by one inch, then a two-pound weight would stretch it two inches, and so on. The same experiment tried with a watch spring or with wire, wood, or any ‘springy body’ would point towards the same rule of nature, ‘that the force or power thereof to restore itself to its natural position is always proportionate to the Distance or space it is removed therefrom’. Knowing nothing about the electrical and chemical forces that bind atoms together, Hooke had nevertheless devised a law of springiness which is fundamental to modern engineering, and thereby established the science of the strength of materials and the behaviour of solids under stress . . . To explain the behaviour of springs Hooke devised a new theory of the nature of matter . . . Congruous bodies consisted of vibrating particles of the same or a ‘harmonious’ magnitude and velocity, and because of this they clung together, defending their space and (if they were solid) their shape from the invasion of other particles, and resisting the tendency to disperse into their surroundings. The shape and size of any body, and therefore the space it occupied, was determined by the vibrating movements of its particles and by the constraining movement and pressure of the ‘ambient fluid’ – the air or aether – that surrounded it . . . the theory that hard particles were in random motion over relatively large distances, colliding with each other without loss of energy, and exerting a greater outward pressure the more they were compressed together, was Hooke’s, and was drawn from his work with Boyle on the compression of air . . . Only with the work of James Joule in the 1840s, and Rudolf Clausius and James Clerk Maxwell in the 1860s, did the kinetic theory of heat and matter achieve general acceptance, and then its origins were traced back 120 years to Bernoulli, not 180 years to Hooke’s pioneering work on elasticity” (Inwood, The Man Who Knew Too Much (2002), pp. 273-6).

The Stanitz copy (in modern binding and with 2 plates cropped) made $1430 in 1984, and the Andrade copy $896 in 1965.

4to, pp. [ii], 56, with 3 engraved plates, woodcut diagrams in text (small repair to upper outer corner of first 2 leaves, not affecting printing, first and last leaf soiled). Eighteenth-century marbled wrappers.